Maths Exam
| 1. | Solution of linear equations
|
| 2. | Differentiation A
|
| 3. | Differentiation A
|
| 4. | Differentiation B
|
| 5. | Integration
|
| 6. | Integration
|
| 7. | Consider the following differential equation: $$ 4\frac{d^2y}{dx^2} - 4\frac{dy}{dx} + 2y = 0 $$
|
| 8. | Consider the following series: $$ \sum_{j=0}^{15} -6\left(j + 6\right) $$
|
| 9. | Solution of linear equations
|
| 10. | Differentiation A
|
Other Articles:
|
Atomistic Simulation of MetalsThis presents an interactive simulation of atoms making up a nanoscopic particle of metal. |
AsciishipMy latest (early 2018) thing is just a "normal" game: no real physics. It's just a game. |

|
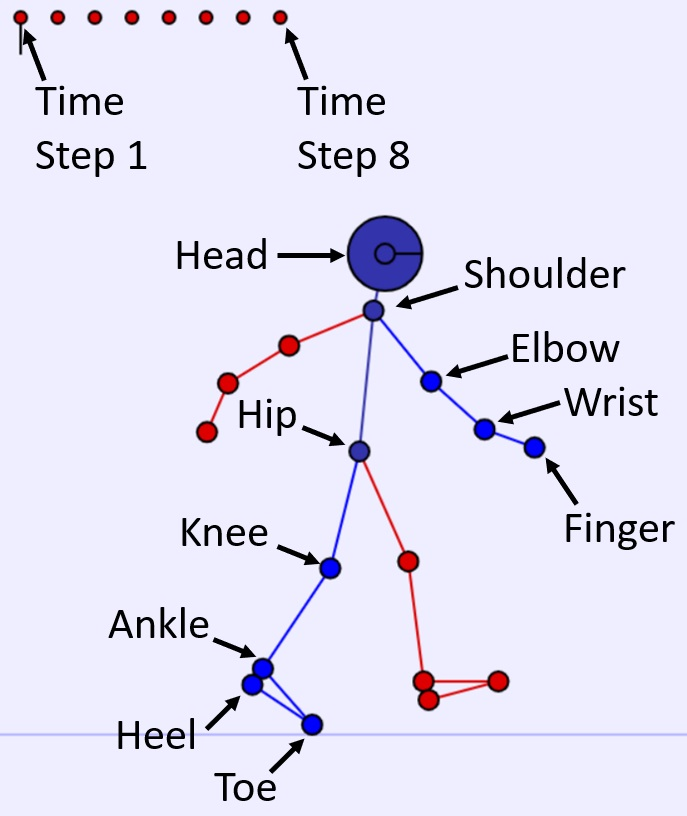
BodyWorks: Kinematics ToolA page with a javascript application where you can set body positions, calculate joint angles and animate human motion. |
|